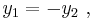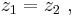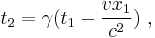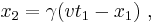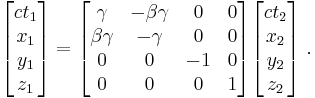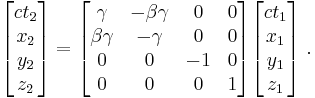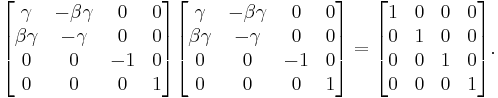Lorentz transformation under symmetric configuration
In physics, the Lorentz transformation converts between two different observers' measurements of space and time, where one observer is in constant motion with respect to the other.
Assume there are two observers  and
and 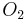 , each using their own Cartesian coordinate system to measure space and time intervals.
, each using their own Cartesian coordinate system to measure space and time intervals.  uses
uses 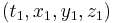 and
and 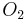 uses
uses 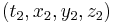 . Assume further that the coordinate systems are oriented so that the
. Assume further that the coordinate systems are oriented so that the 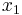 -axis and the
-axis and the 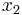 -axis overlap but in opposite directions. The
-axis overlap but in opposite directions. The 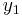 -axis is parallel to the
-axis is parallel to the 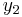 -axis but in opposite directions. The
-axis but in opposite directions. The 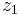 -axis is parallel to the
-axis is parallel to the 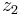 -axis and in the same direction. The relative velocity between the two observers is
-axis and in the same direction. The relative velocity between the two observers is 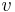 along the
along the 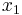 or
or 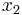 axis.
axis. 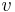 is defined as a positive number when
is defined as a positive number when  sees
sees 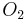 sliding in the direction of
sliding in the direction of 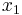 . Also assume that the origins of both coordinate systems are the same. If all this holds, then the coordinate systems are said to be in symmetric configuration.
. Also assume that the origins of both coordinate systems are the same. If all this holds, then the coordinate systems are said to be in symmetric configuration.
In this configuration, frame 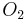 appears to
appears to  in the identical way that frame
in the identical way that frame  appears to
appears to 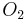 . However, in the standard configuration, if
. However, in the standard configuration, if 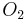 sees
sees  going forward then
going forward then  sees
sees 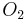 going backward. This symmetric configuration is equivalent to the [Lorentz transform#Lorentz transformation for frames in standard configuration|standard configuration]] followed by a mirror reflection of the x and y-axes. For the stationary
going backward. This symmetric configuration is equivalent to the [Lorentz transform#Lorentz transformation for frames in standard configuration|standard configuration]] followed by a mirror reflection of the x and y-axes. For the stationary 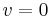 case, this reduces to only the reflections, whereas the standard form reduces to the identity transformation.
case, this reduces to only the reflections, whereas the standard form reduces to the identity transformation.
The Lorentz transformation for frames in symmetric configuration is:
where 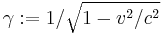 is the Lorentz factor.
is the Lorentz factor.
The inverse transformation is:
The above forward and inverse transformations are identical. This offers mathematical simplicity.
In matrix form the forward symmetric transformation is:
where 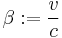 .
.
The inverse symmetric transformation is:
A single transformation matrix is used for both the forward and the inverse operation.
As expected: